Experiment of The Month
How High is the Moon?
The MU Physics Department does not claim to have invented these labs. The origin of these labs is currently unknown to us. Our labs do not have written instructions. In keeping with this spirit, the description given here will be brief and general. The intent is that each performance of the lab will be unique; in each nature will reveal a slightly different face to the observer.
This past month we measured the distance from the surface of the earth to the moon. Although the answer can be looked up, it is important to know how easy it is to measure that distance.
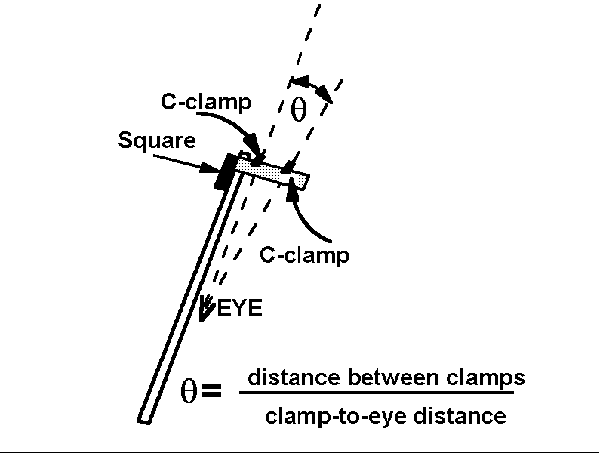
The basic measurement is the determination of the angle between the moon and a star (or planet) "near" to it in the sky. (When the moon is half-full, it gives evidence that the stars and planets are farther away by hiding them behind its "dark half.") The angle is measured with the device shown, by lining up the moon with one c-clamp and the star with the other.
The measurement of that angle leads to a measurement of distance to the moon when it is repeated, at the same time, by a distant observer. The two observers in this case were placed in at Millersville, PA (Dr. Dooley of the MU Physics Department) and Ganado, AZ (H. Pertusio of the Ganado School on the Navajo Reservation). They measured different angles, and the difference in the measurements reveals the distance to the moon.
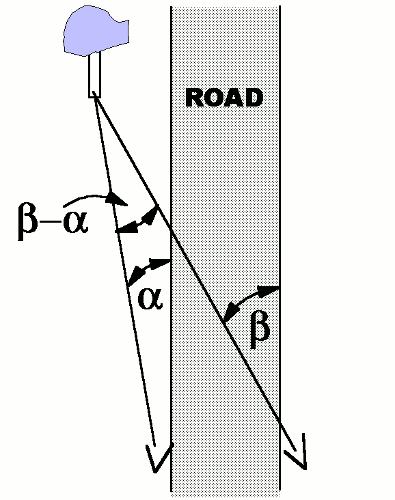
The figure illustrates a terrestrial version of such a parallax measurement. The two parallel sides of the road define a convenient reference direction for two observers, one on each side of the road. The angle between the line to the tree and the road is different for the two observers. The reader can show that difference between the two measured angles is the same as the angle subtended by the two observers, with the vertex at the tree.
Knowing the separation of the observers, and the angle that they make with the tree as vertex, allows calculation of the distance to the tree. In the case of the moon measurement, the line from a distant star to each observer replaces the two edges of the road as a reference direction.
(There are of course two lines from star to the two observers, and those two lines are not really parallel. However, if the star is far away, the lines are nearly parallel. For this measurement, the angle between those two lines is small enough that we consider it to be zero.)
In the present case, the observers are separated by about 1800 (pardon the expression) miles. We assume that the two observers, the moon and the reference star all lie in the same plane. This is not correct, but they lie close to the same plane. The difference in measured angles (moon-to-star with observer at vertex) gives the observer-to-observer angle, with the moon at vertex.
It is important that the angles be measured at the same time, because the moon "falls behind" the starry background. We see the stars rise above the eastern horizon at almost the same time each night in a given month. On the other hand, the moon rises about an hour later each night: Every night it is about fifteen degrees further away from a star to the west of it. If we measure at different times, we must correct for this drift through the stars.
The measurements were synchronized by email, exchanged quickly, as shown in the log below. The final result for the distance to the moon from the surface of the earth was about 100,000 miles, with an uncertainty of about 50,000 miles. Although some data were regarded as better than others, in the end there was no justification for discarding any of the data. The results quoted are for the average of 3 measurements for two different reference stars, for a total of 6 measurements.
It is worth noting that although there was a fairly large variation in the angles and their differences, the differences always had the same sign: The angle between the moon and a star to the west was always larger in Arizona than in Pennsylvania. More accurate measurements are possible, but the point is that the measurements can be done with simple equipment. The internet is not necessary, but makes a nice way to verify that the measurements are done at the same time.
Moon Measurement Log
10/18/99 5:17:51 PM Eastern Daylight Time From: Jwdooley To: detisue@hotmail.com Sent on: AOL 4.0 for Windows 95 sub 26 In a message dated 10/18/99 11:10:36 AM Eastern Daylight Time, detisue@hotmail.com writes:
<< How do I get the angular measurement from your measuring tool? >>
Set the two c-clamps a centimeter apart or so, and move your eye back along the stick until one is one the star when the other is on the moon. (1 cm)/(eye-clamp distance) is the angle in radians. You may have to adjust the 1 cm depending on how far the star is from the moon.
Can you email me about 10:30? John
*************10/18/99 10:39:24 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio) To: Jwdooley@aol.com
I am here now. H
*************10/18/99 10:40:31 PM Eastern Daylight Time From: Jwdooley In a message dated 10/18/99 6:48:17 PM Eastern Daylight Time, detisue@hotmail.com writes:
<< I just found out that we will be moving in November to a brand new 3-bedroom, 2-story townhouse. I am so excited! :-) >> Good for you!. Right downtown.. I don't have many stars visible - a little hazy. There is a pair to the west of the moon, the upper one farther to the west than the lower one. See those? John
*************10/18/99 10:46:02 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
We just checked and there is NOTHING within a reasonable distance from the moon (and we have a perfectly clear night). The moon is too bright. There is a very bright planet in the sky but it is not close enough to the moon to use as a reference. Heidi
*************10/18/99 10:49:42 PM Eastern Daylight Time From: Jwdooley
Did you see the pair to the west? To get the angle I had to separate the c-clamps by about 10 cm and look back only about 50 cm. (rough numbers - I don't want to bias you.) () * * Something like that with () being the moon John
*************10/18/99 10:54:21 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
I see them both. Which will be our reference?
*************10/18/99 10:57:09 PM Eastern Daylight Time From: Jwdooley
Let's measure both. We should agree on the difference between two angles (moon to low star and moon to high star.) That will be a good test of our accuracy. I'm off to take the measurements now. 10:57 here John
*************10/18/99 11:02:39 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
closer star: 0.174 other star: 0.220 difference: 0.46
*************10/18/99 11:07:09 PM Eastern Daylight Time From: Jwdooley
Measuring with the center of the moon on the center of the screw (It's about .6 cm wide) I get .168 for the near star .198 for the far star so my difference is .03 Thinking... John
*************Date: 10/18/99 11:08:29 PM Eastern Daylight Time From: Jwdooley
We're not good enough. Want to remeasure one time? John
*************10/18/99 11:09:23 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
I will measure again if you would like. Shouldn't we average the results of several measurements? :-) Heidi
*************10/18/99 11:11:04 PM Eastern Daylight Time From: Jwdooley
Sounds good. I'll take two more at each star, still with the left pin in the middle of the moon. It's 11:11 as I go out. See you in a few minutes. John
*************10/18/99 11:15:38 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio) To: Jwdooley@aol.com
measurement 2 closer: 0.182 other: 0.196 difference: 0.014
*************10/18/99 11:24:40 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
measurement 3 closer: 0.180 other: 0.206 difference: 0.026 average closer: 0.179 average other: 0.207 average difference: 0.028 The farther star is much fainter than the closer star and my measurements with the closer star are more consistent. If you want to use anything from tonight's measurements, I recommend using the average measurement using the closer star. Heidi
*************10/18/99 11:27:38 PM Eastern Daylight Time From: Jwdooley
closer .159 farther .186 difference .027 closer .159 farther .192 difference .033 Thinking John
*************10/18/99 11:30:58 PM Eastern Daylight Time From: Jwdooley
I think we have it. We agree within .005 on the second two measurements, and I'll cast out the first because we disagreed by more. Looks like a difference in angle of about .02 radians with yours bigger than mine. More thinking John
*************10/18/99 11:35:25 PM Eastern Daylight Time From: Jwdooley
Well, it looks like the moon is 1.5 x 10^5 miles away. Book says about 3 x 10 5 Not bad !! john
*************10/18/99 11:37:33 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
What now? Heidi
*************10/18/99 11:41:11 PM Eastern Daylight Time From: Jwdooley
I'm going to bed with a big smile. I'll package up our conversation for presentation to my class, and send you a copy if you want. I'll smile about my moon and you can smile about your *town house.* I can't believe it worked first try, and with stars so far from the moon. If a planet gets near on a clear night, maybe we can try again. The NASA grant folks want people to use their data base. I'll see if there is a way to tie our measurements to their data. I thought you might be able to use some observing or computer equipment. Think about what you might ask for. See ya! John
*************10/18/99 11:43:33 PM Eastern Daylight Time From: detisue@hotmail.com (Heidi Pertusio)
Good night, happy professor! Heidi PS. I'd love a copy of what you present to your students. Thanks!
*******************************************************